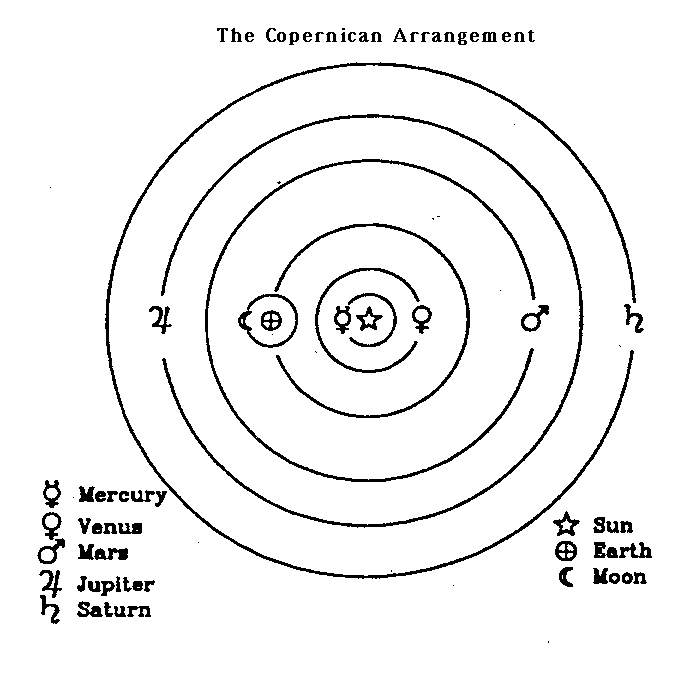
 (photo) Nicholas Copernicus was a Polish-German clergyman. Most famous
for developing a Sun-centered. Did need epicycles and reasons not accepted
universally.
(photo) Nicholas Copernicus was a Polish-German clergyman. Most famous
for developing a Sun-centered. Did need epicycles and reasons not accepted
universally.
Early physics was mostly concerned with describing motion. The ancient Greeks had a well developed concept of motion. This was codified in Aristotle's books "Physics" and "Caelo" (Astronomy - literally sky or heavens). A brief summary of Aristotle's Physics (click on previous two words) is found at this connection and in the text book. It is a physics based upon observation, aethestics and common experience.
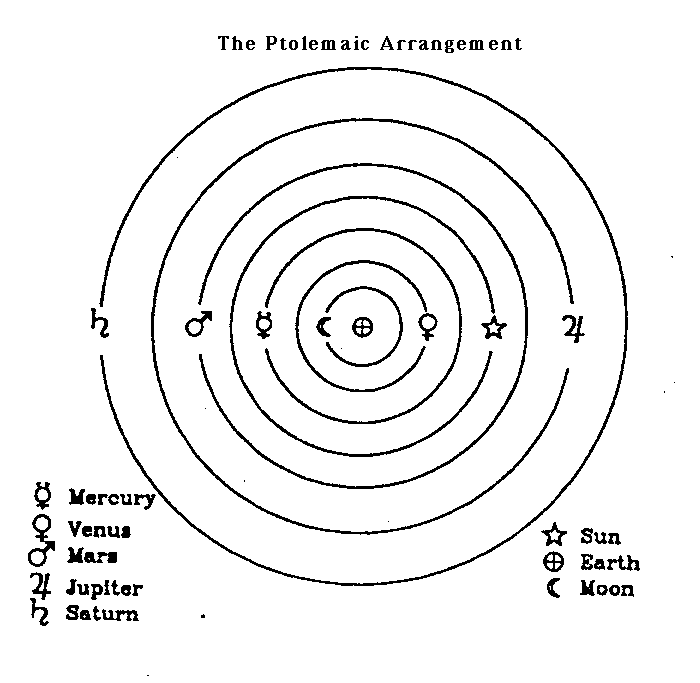
An important part of describing motion was describing the behavior of the heavens. The Greeks realized that the motion of nearly everything observed in the sky could be described very simply. All the stars were fixed relative to each other and seemed to rotate about the Earth each day (24 hour period). The dark night sky reminded them of the inside of a black bowl with bright lights attached. Thus the idea that all the stars were fixed upon a sphere which rotated about the Earth once per day.
Then there were other special cases, the Sun, the Moon, and the planets (a Greek word meaning wanderer). Clearly these too could be explained by simple uniform circular motion. Though the Greeks worked out a number of ways to describe the motion of the heavens, including one in which the Sun was at the center which was a Copernican view, the model that was accepted was the one which came to be call the Ptolemaic model. It was chosen on the basis of the accepted physics and observations - particularly the apparent lack of stellar parallax. This model held sway longer than any other scientific model in history even with the constant attention and observations of astronomers through out the millenia.
For all those centuries, until Johannes Kepler, astronomers, mathematicians, and scientists tried to describe the motions of the planets based upon three assumptions about planetary motion
Nicholas Copernicus (1473-1543)
 (photo) Nicholas Copernicus was a Polish-German clergyman. Most famous
for developing a Sun-centered. Did need epicycles and reasons not accepted
universally.
(photo) Nicholas Copernicus was a Polish-German clergyman. Most famous
for developing a Sun-centered. Did need epicycles and reasons not accepted
universally.
Tycho Brahe (1546-1601)
Tycho Brahe was one of the greatest astronomical observers of all time.
Clearly the most outstanding before the use of telescopes.
Tycho observed 11 November 1572 a supernova in the constellation Cassiopeia.
The cosmology of the day was that the heavens, since they were created
by God, were perfect and unchanging. It was inconceivable to an Aristotelian
that a new star could appear in the immutable heavens (8th shell).
There had been a previous very bright supernova in 1006 AD
so bright as to rival the moon followed a fifty years later, 1056 AD
by the Crab supernova. These supernova were recorded by astronomers all over
Asia and reputedly in the Americas.
However, no European astronomer reported it.
The only remarks seem to be about a bright comet which since the time
of Aristotle had like meteors (hence the name from meteorology) thought
to have been atmospheric phenomena.
The astronomers of Tycho's day refused to admit that it was a star
(supernova) and in frustration Tycho is reputed to have said,
"O crassa ingenia, O coecos coeli spectatores." - translates "O thick
wits, Oh blind watchers of the sky."
Tycho Brahe also observed the comet of 1577. Most astronomers used the
method of Regiomontanus (1436-1476) to determine the parallax and thus the
location
of the comment. Regiomontanus' method assumed
(1) No intrinsic motion
(2) no uncertainty in the
timing of observations
(3) the object could be
observed on the meridian
Tycho Brahe not only made better observations but he developed a better
method for determining the position of the comet from the observations.
Tycho was able to show that the comet must pass through (and cracked) the
crystalline spheres.
This is a key point in the progress towards a more accurate
description of the planets motion which was primarily
a result of Tycho Brahe's own high quality observations.
Johannes Kepler (1571-1630)
Johannes Kepler, using the data from Taco Brahe, having set to the task of working on describing the orbit of Mars, came to realize the older assumptions were wrong and developed three new laws of planetary motion.
Galileo Galelei (1564-1642)
Modern science techniques
1609 Galileo turns telescope to the heavens and discovers:
1. thousands upon thousands
of stars invisible to the naked eye
2. Surface of the Sun is
not perfect, has spots.
3. There are mountains
and craters on the Moon. It too is not perfect.
4. Venus goes through phases
just as the Moon does -> Copernican model explains better.
5. Rings of Saturn
6. Could resolve that image
of planets but not stars. Also could see parallax for planets but not for
stars. Stars must be very, very far away.
7. Nebulus part of the Milky
way was actually stars
8. Four moons of Jupiter
- moons clearly orbit Jupiter and not the Earth - so could other things
orbit the Sun and other planets.
1610 Galileo publishes these in the Starry Messenger.
Galileo became convinced that the Copernican model was actually
the way the heavens moved and realized that the Physics of Aristotle
stood in the way of its acceptance as much as the Catholic Church.
Galileo had other motivation and perspectives that led him to proceed to develop methods of doing science that are the foundations of modern science and these led to a new understanding of motion.
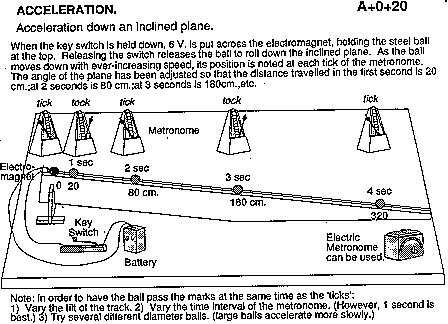
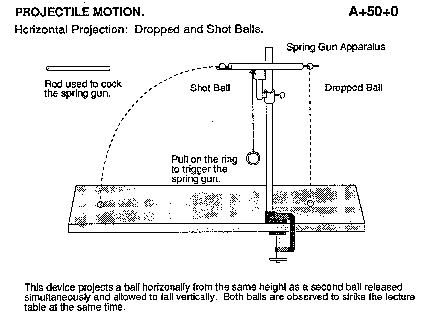
Galileo studied motion carefully.
Now I crumple up the paper into a tight ball. It is the same weight as it is the same paper. I release the paper and ball simultaneously and they hit the table top essentially simultaneously. Galileo realized that air resistance was a major extraneous factor and that if one idealized an experiment without air (in vacuum) then objects would fall at the same rate.
D = a t 2 / 2
were a is the acceleration
due to gravity usually denoted by g .
The factor of two comes from the fact the ball starts are rest
and so at time t the ball has
speed v = a t so that its average
speed is v average = v/2 = a t / 2
and the distance traveled is
D = v average t = a t 2 / 2 .
Issac Newton (1642-1727)