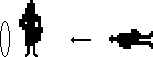
Experiment 6: Einstein's wife wants him to jump through
hoops, but he wonders if anything strange will happen with regard to lengths
that are perpendicular to his direction of motion.
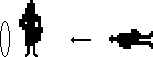
Conclusion: Let us suppose that when an object moves that there is a contraction of length in the direction that is perpendicular to its line of motion. Then the diagrams below will show that this assumption leads to a contradiction.
By this assumption, if the hoop considers itself as standing still, then
Einstein shrinks in the direction perpendicular to his motion and has no
trouble going through the hoop.
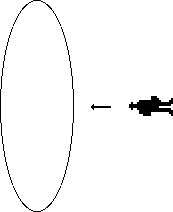
On the other hand, if Einstein considers himself as standing still and
the hoop as moving toward him, then by our assumption he would see the hoop
contract, and conceivably, he might then be unable to pass through the hoop.
This is a contradiction, though, since we can't have Einstein both passing through and not passing through the hoop. The assumption that leads to this contradiction is that lengths perpendicular to our line of motion will contract. An assumption that these lengths would expand leads to a similar contradiction. Thus, we can only conclude that there will be no change at all in lengths that are perpendicular to our line of motion. The only lengths that change are those that are parallel to our line of motion, as we showed in the previous experiment.