
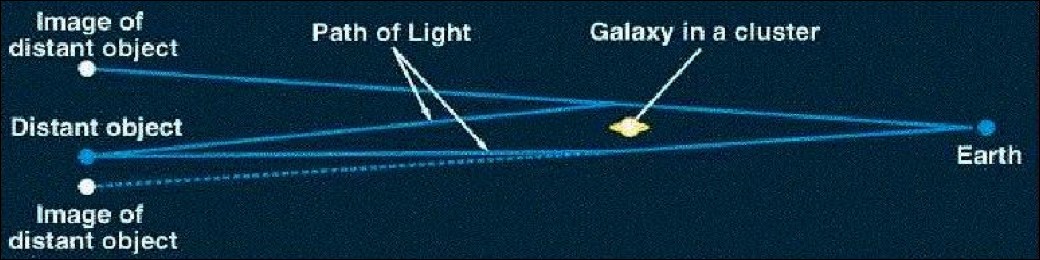
What Causes Gravitational Lensing?
Gravitational lensing can be explained by Einstein's general theory of
relativity. Galaxies, which are very massive objects, act on spacetime
by causing it to become curved. This curved spacetime then influences
particles in its vicinity, affecting their motion. For example, photons
travel the path of minimum spacetime. What appears to be the shortest path
in flat spacetime is not the shortest path in curved spacetime. For this
reason, photons passing a massive object take curved paths. Differential
curvature results in distorted and magnified background images. In typical
weak lensing, these effects are on the order of a few percent. Since
galaxies have random rotation and inclination, shear effects must be
determined by statistically preferred orientations.
What Effects Should We See
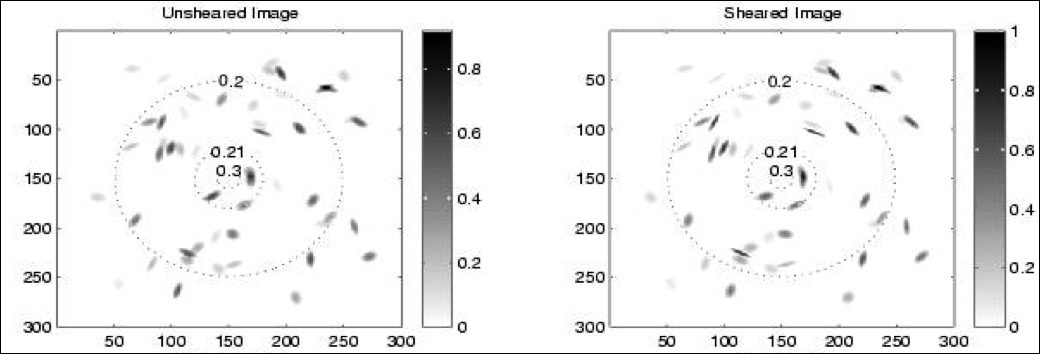
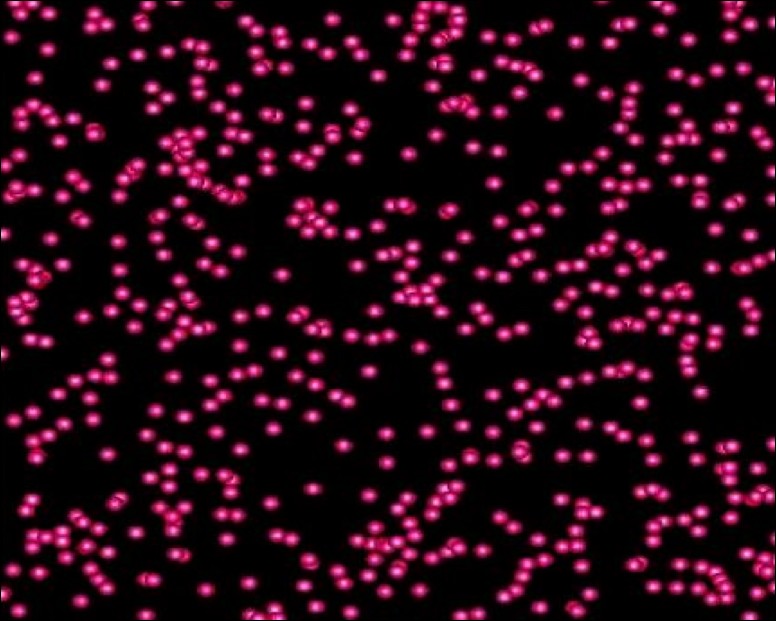
Unlensed
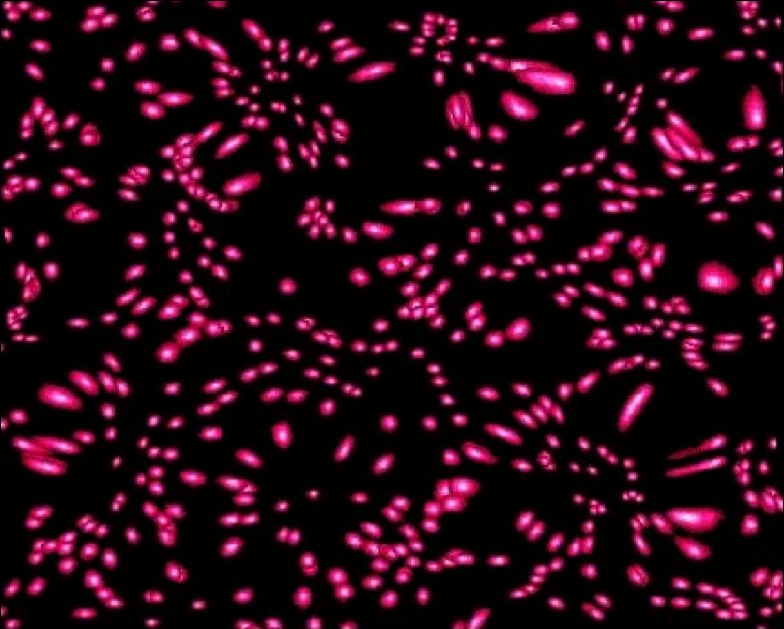
Lensed
Sources of Error
The primary source of error in lensing measurements is due to the
convolution of a point spread function (PSF) with your lensed image. A PSF
is a distortion due to several factors, primarily imperfect optics, as
well as atmospheric smearing in ground-based studies or thermal breathing
in space based observations. There are also numerous other contributions due
to physical limitations. There are other sources of error removed from the
optics as well, such as centroid determination for ellipticity
calculations, Poisson, and statistical bias/errors due to orientation
assumptions and limited galaxy magnitudes.
What Do Noisy Images Look Like?
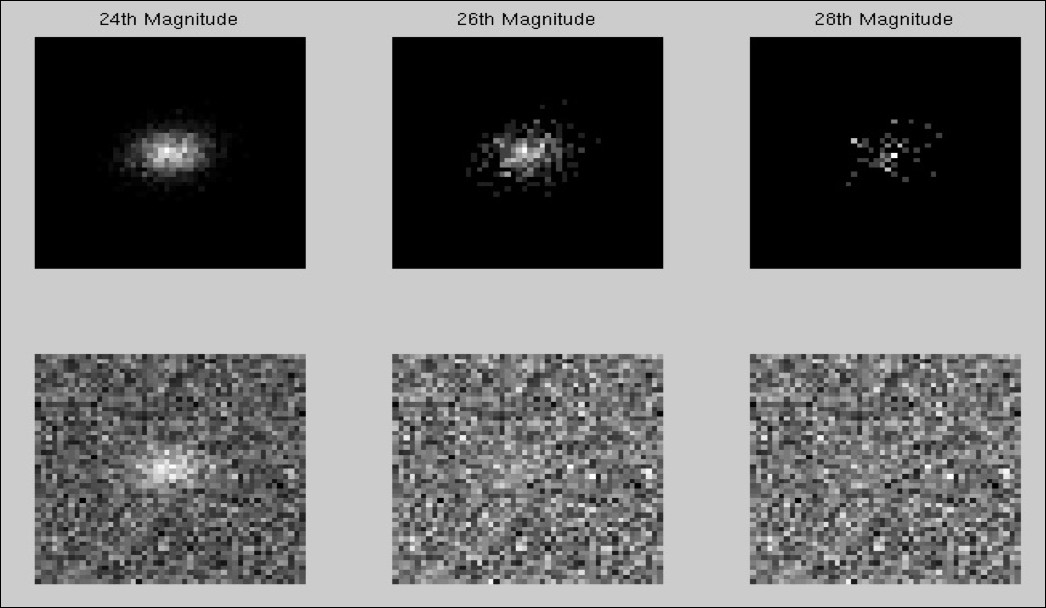
Method I: Shapelets
"Shapelets," developed by Refregier (2001), is a method which has been
applied to analyzing images and accurately measuring weak shear. This is
accomplished by decomposing individual galaxy images into a complete
orthonormal basis set consisting of weightier Hermite Polynomials. The
basis functions are perturbations around a circular, 2-dimensional
Gaussian distribution, and are eigenstates of the 2-dimensional Quantum
Harmonic Oscillator (QHO). The shapelets method has notable advantages
over other methods. First, it is invariant under Fourier transformations,
which allows accurate Correction of the PSF through a deconvolution
process. It is also possible to determine the total flux, centroid, and
the radius of a lensed object, of which the accurate measurements provide
a significant advantage in measuring shear.
Method II: Kaiser, Squires, and Broadhurst (KSB)
The KSB method measures the ellipticity of a galaxy image. In the weak
lensing regime, the shear is proportional to the ellipticity, which can be
measured by determining the quadropole moments of an image. To reduce
noise, the signal is convoluted with a weighting, typically a gaussian.
KSB's primary advantages are its mathematical ease and relatively simple
implementation. However, KSB also requires an independent correction for
the PSF, generally difficult, and since KSB measures ellipticity, it is
necessary to introduce a statistical correction factor based upon assumed
average orientations to obtain the shear.
References
Kirsten Howley, Javier Guedes, Daniel Raburn, Chris Sitzer, Prof. Jodi
Lamoureux(Cal Poly San Luis Obispo), & Prof. George Smoot(UC Berkeley),"Weak Gravitational Lensing by Clusters of Galaxies"
Lectures on Gravitational Lensing-Bartlemann & Narayah; 2004,astro-ph/9606001
Bartelmann, M.2002, astro-ph/0207032
Kaiser, N.,Squires,G.,Broadhurst, T., 1995, astro-ph/9411005
Refreiger, A, 2001, astro-ph/0105178
Refregier, A., Bacon, D., 2001, astro-ph/0105179